Optimizing Display Other Formats:      PDF - 375k

Volume 2 Issue 1 (2003)        DOI:10.1349/PS1.1537-0852.A.263

Note: Linguistic Discovery uses Unicode characters to represent phonetic symbols. Please see Optimizing Display for requirements to accurately reproduce this page.

Iambic Feet in Paumari and the Theory of Foot Structure^[1]

Daniel L. Everett

University of Manchester

This paper analyzes stress and moraic constituencies in Paumari, an endangered language of the Arawan family of the Brazilian Amazon. It argues that Paumari feet are quantity-insensitive iambs, built from right-to-left within the prosodic word. Both of these latter claims are theoretically important because they violate some proposed universals of foot structure. The paper also discusses more general implications of the Paumari data for theories of foot size and shape, proposing two constraints on foot size, Foot Maximality and Foot Minimality, to replace the less fine-tuned constraint Foot Binarity.

1. Introduction

The principal purpose of this paper is to describe the unusual stress placement facts of Paumari, a member of the Arawan linguistic family. All of the Arawan languages are spoken near the Purus river in Western Amazonas state, Brazil. Like the other Arawan languages, Paumari is highly endangered. There are only a few hundred speakers of Paumari, and most of them speak Portuguese increasingly, even among themselves (although Paumari is spoken more often than Portuguese, and children still grow up speaking Paumari). It is, therefore, extremely urgent that we document this language now, before the lessons it has to teach us are lost. The languages belonging to the Arawa family are: Paumari, Madi (comprised of three dialects, Yarawara, Yamamadi, and Banawá), Deni, Kulina (these latter two are members of a subfamily, Madijá), Suruwahá, and Arawá (extinct for several decades). See Buller, Buller, and Everett (1993) and, especially Dixon (2000) for details on Arawan. Secondarily, the paper discusses the theoretical implications of this description for some widely assumed theories of stress and foot theory. For concreteness, the paper adopts an Optimality-Theoretic approach to the analyses, though there is no intent to thereby endorse any particular theory. The paper’s empirical contribution is the description of an unusual stress system from an endangered Amazonian language. Theoretically, the paper contributes to our understanding of the interactions between foot structure, syllable shape, and word-size. It concludes that some widely-accepted theories of iambic feet are incorrect.

The paper is organized as follows. Section two describes the basic features of Paumari stress placement and gives the Paumari segmental inventory. Section three offers a more detailed OT analysis of prosodic constitutents in Paumari – words, syllables, and feet. It also offers an analysis of variations in stress placement in reduplicated forms. It considers and rejects a trochaic counteranalysis in the final subsection, clearing the way to draw a number of theoretical conclusions about iambicity from the Paumari facts. In section four these implications are considered in more detail. The paper concludes with a summary of its major findings.

2. An overview of stress placement in Paumari

2.1. Phonemic inventory

The Paumari segmental inventory is about average size, as Amazonian languages go, with 23 phonemes. But this relatively small inventory includes some areally unusual phonemes, in particular two aspirated voiceless stops and two voiced implosives. Dixon (2000) provides a clear overview of Arawan segmental phonology and a partial reconstruction of proto-Arawan phonology, including Paumari data in his reconstruction.

The phonemic inventory of Paumari is (the orthographic symbol is in bold followed by the phonemic symbol): 'b /∫/ voiced glottalized implosive bilabial stop; 'd /Î/ voiced glottalized implosive alveolar stop; b /b/ voiced bilabial stop; d /d/ voiced alveolar stop; g /g/ voiced velar stop; j /dʒ/ voiced alvelopalatal affricate; p /p/ voiceless bilabial stop; t /t/ voiceless alveolar stop; th /t^h/ voiceless aspirated alveolar stop; k /k/ voiceless velar stop; kh /k^h/ voiceless aspirated velar stop; tx /tʃ/ voiceless alvelopalatal affricate; f /f/ voiceless labio-dental fricative; s /s/ voiceless grooved alveolar fricative; h /h/ voiceless glottal fricative; m /m/ bilabial nasal stop; n /n/ alveolar nasal stop; r /ɾ/ voiced alveolar flap; v /w/ voiced labiovelar approximant; ' /ʔ/ glottal stop; i /i/ high front close vowel; o /o/ back mid rounded vowel; a /a/ central low vowel.

2.2. Basic stress facts

For the moment, let us assume that Paumari syllables are always of the shape CV or CVV and that they are always monomoraic. This enables us to state the preliminary generalizations about Paumari stress placement in (1) and (2):

These generalizations are illustrated in (3):

In (3a) there is only one, primary, stress in the word. This is because the word is less than three moras in length, activating the qualification clause in statement (2). The same is true of (3b). Notice that the examples in (3) show that CVV syllables are treated like CV syllables for stress placement, regardless of their position in the word. This is seen by the fact that stress skips them entirely when they are to the left of an odd-numbered mora. Since all syllables in (3) are impressionistically the same length and behave the same with regard to stress placement, I have analyzed them all as monomoraic. It is also relevant to note that when one of the monomoraic CVV sequences in stressed, stress goes on the most open or sonorant vowel, whereas sonority does not influence stress placement in the words in (4).

As just stated, CVV syllables are normally monomoraic and when they are stressed, their most open/sonorant vowel bears stress. However, there is an important class of exceptions to this, namely, monosyllabic CVV words (there are no CV monosyllables in the language), as shown in (4):

The words in (4) are stressed as the bimoraic words in (3a) and (3b). And the words in (4) are impressionistically of the same duration as the words in (3a) and (3b), i.e. other bimoraic words. The CVV sequences of (4) are obviously and significantly longer in duration than the CVV sequences in (3). Also, the CVV sequences in words like those (4) are always stressed on the final mora, regardless of the sonority/openness of its associated vowel, unlike the CVV sequences in (3), where the most open/sonorant vowel bears the stress.

The duration and stress contrasts between the CVV sequences in (3) and (4) are due to the fact that in Paumari no word may be less than two moras in length. Monosyllabic words, composed of syllables that would otherwise be shorter than two moras, are bimoraic in duration, evidenced by their perceived length and stress placement, thus satisfying this constraint on minimal word size. This constraint is formalized in section three below. All Arawan languages except Deni (see Everett (1995), Buller, Buller, and Everett (1993), and Ladefoged, Ladefoged, and Everett (1997)) manifest this same bimoraic minimal word constraint. Further examples of stress placement in Paumari are given in (5)-(9) (where ` = secondary stress):

I formalize this analysis in section three. I restate this rule slightly, using syllables rather than vowels as the basic stress-bearing unit.

3. Prosodic constituents within the word

3.1. Introduction

Phonological theory over the past decades has accepted and developed ideas from centuries of work on poetic meter and literature. Namely, various sound groupings above the segment exist and are vital to the understanding of how native speakers use and perceive their language. Modern prosodic theory has enriched our understanding of these groupings, labelling them and showing that languages carefully constrain the relative sizes of the different groupings. I am here concerned with three groupings: syllables, feet, and words. The generalization is that the maximal size permitted on one level is the minimum size permitted on the next level up.

The sizes are given in (10), with argumentation to follow:

Each generalization is justified in the following subsections.

3.2. Word size

In all Arawan languages (except for Deni, see Everett (1995)), phonological words are never less than two moras in length. Paumari fits this pattern. Hypothetical, negative evidence is given for this generalization in (11) and positive evidence in (3) and (4).

There are no prosodic words in Paumari like the hypothetical forms in (11):

(11) *pi, *sa, *du, etc.

Positive evidence for this constraint is found in the weight of vowel sequences in words like (4) above vs. the vowel sequences in (3), repeated here:

Only the vowel sequences in words like (4) are ever bimoraic. Vowel sequences are strictly monomoraic elsewhere, e.g. in (3). This contrast also shows that the length of the diphthongs in (3) cannot be due merely to their position within the word. We explain both examples like (11) and the contrast between (3) and (4) by positing the constraint in (12) below, which is found in other Arawan languages:

(12) Minimal Word Size (WdMin: *[m]): Words in Paumari are at least two moras in length.

We return to this constraint below and show its effects in words like (4) more precisely. First, however, we must consider a constraint on syllable size which interacts with (12).

3.3. Syllables and syllable size

Standard arguments for syllable shape and size are found in a given language’s phonotactics (Ewen and van der Hulst (2001:122ff) and stress placement facts (Hayes (1995)). Such arguments have been buttressed recently in work by Gerfen (2001:185ff), who argues that direct phonetic licensing of segments (and thus phonotactics), as advocated in Steriade (1997), is inadequate and that phonotactics still require reference to syllables (though cf. Blevins (2003)).

Here are the phonotactic observations I would like to explain:

Observation (13a) is based on the absence of Paumari words like the hypothetical forms in (14):

Consider how (13b) accounts for the grammaticality contrasts in (15)–(21). The ungrammatical examples are stressed by allotting one mora per vowel. The grammatical examples are stressed by allotting one mora per vowel sequence/syllable.

Words such as the hypothetical forms in (22) are also missing from my corpus and in fact are predicted not to occur by the analysis I have proposed above. That is, there are no long vowels in Paumari in my analysis.

The remaining observations are based on facts already illustrated above. Reasoning abductively, what assumptions might we make about the observations in (13) above to render them unsurprising? I suggest the proposals in (23):

The type of analysis I have been assuming so far assumes a CV phonotactic arrangement and alternating stress pattern in Paumari words, with deviations from this simple basis forced by more important (to Paumari) constraints on word shape. This kind of approach can be neatly formalized in Optimality Theory (OT). In an OT analysis of a language, we must identify the relevant operative constraints in a language and rank them. Any constraint can be violated in order to obey a more highly-ranked constraint.

The proposals in (23) can thus be translated into the constraints in (24), ranked as in (25). The interplay of these constraints is shown in Tableaux 1-3.

(25) Max IO_seg,, Dep IO_seg >> WdMin >> SylMax >> Onset

To fully appreciate these constraints and their ranking, consider the following tableaux: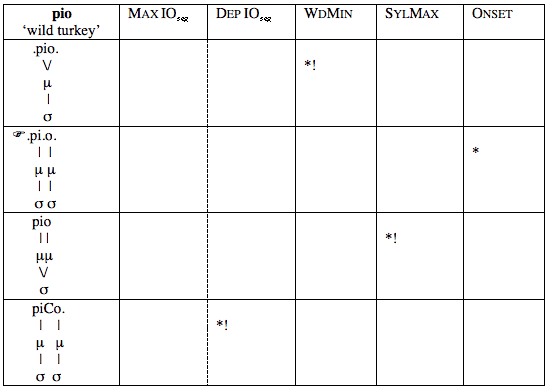

Tableau 1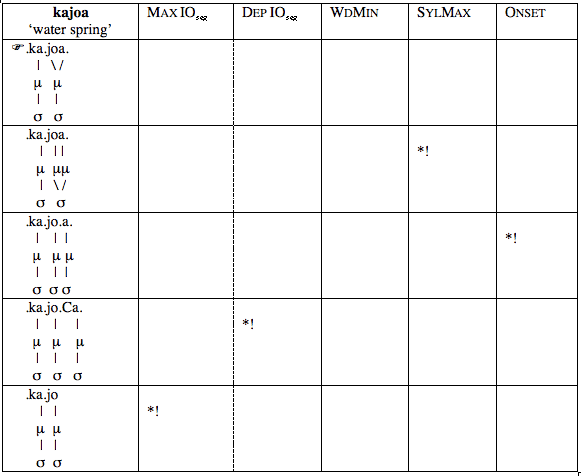

Tableau 2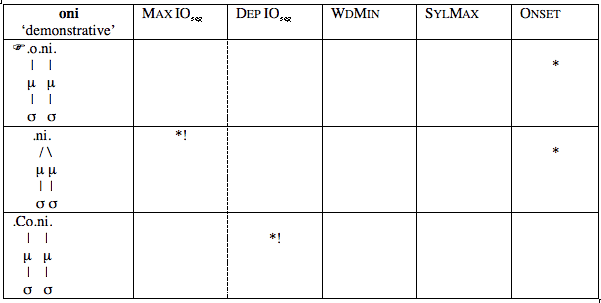

Tableau 3

In the next section, this analysis is formalized somewhat, so that its significance can be better appreciated.

3.4. Foot structure and secondary stress

Since, as shown in the previous section, syllables are always monomoraic, I will make the least controversial assumption (Hayes (1995)) and claim that feet are built on syllables in Paumari. All feet on the lowest layer (secondary stress) are iambic – right-headed, binary groups of syllables:

Moreover, all feet are oriented towards the right edge of the word. To use a process metaphor, they are built from right to left. This is illustrated in (27)-(33).

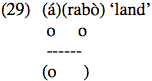
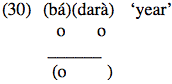
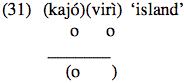
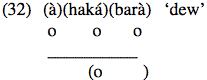
As was the case with syllable structure, so too with stress-placement, we can express the facts observed in terms of a small number of constraints, without derivations and without process metaphors. The constraints I wish to propose, fairly standard in OT, are give in (34)-(35):

Right-to-left orientation (in the sense that degenerate feet appear on the left):

(35) Align-L: (PrWd, Ft)

This constraint says that feet ‘prefer’ to line up on the left edge of the word. As Crowhurst and Hewitt (1997) demonstrate, this will place degenerate feet on the left margin of the word.

To ensure that no syllables are left unfooted, even if footing them would violate FtBin, by creating a non-binary foot, the high-ranked constraint in (36) is proposed:

(36) Parse-s: Syllables are constituents of feet.

These constraints are ranked in (37):

(37) Parse-s >> FtBin >> AlignHd >> AlignFt

Illustrative examples are given in tableaux 4 and 5: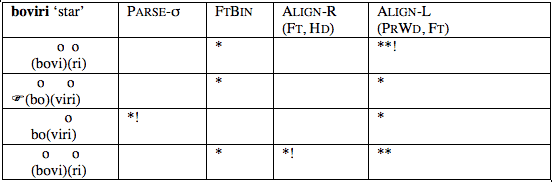

Tableau 4

Tableau 5

3.5. Foot structure and primary stress

Like syllables and secondary stress, primary stress can also be accounted for by ranked constraints, by placing a ‘superfoot’ on the secondary stress feet, a standard approach to primary stress in the generative literature.

Finally, the constraints in (38) correctly determine primary stress placement.

Tableu 6 demonstrates how these constraints work together.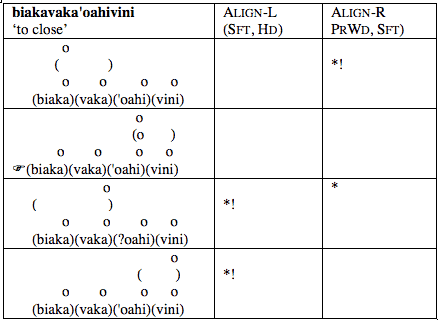

Tableau 6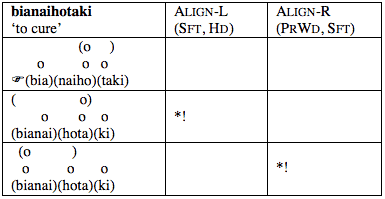

Tableau 7

Our analysis to this point provides a straightforward account of stress in reduplicated forms.

3.6. Reduplicated Forms

Stress on bisyllabic reduplicants occasionally appear to violate the statement of stress placement above (see also Chapman and Derbyshire (1991:349)). This is shown by comparing the prime vs. non-prime examples in (39) below. Both sets of examples are grammatical. In the non-prime example, the reduplicant is stressed on its rightmost syllable, although our analysis predicts it to fall on its leftmost syllable and that there will be no adjacent stresses). In the prime-example, the reduplicant is stressed on its leftmost syllable. Alternatively, however, these forms are handled easily under the proposal that the reduplicant is treated by Paumari grammar as, alternatively, a (at least semi-) separate prosodic unit from the base or as part of the entire reduplicated word (where () = prosodic word and [] = grammatical word).

In the sections which follow I am going to consider the implications of this analysis of Paumari for the widespread belief (see Hayes (1995:80) and Kager (1993, 1995a, 1995b)) that phonetics largely determines foot structure. This claim is known in one form as the ‘Iambic/Trochaic law’, and was first proposed by Hayes (1995:80) and then frequently defended by Kager (1993, 1995a, 1995b, 1997). This ‘law’ is given in (40):

Since my analysis shows that syllable duration plays no role in iambic foot construction in Paumari, (40) is violated. The implications of this violation are taken up in section 4 below. Before considering these implications, however, we need to first consider a non-iambic alternative to the analysis of Paumari stress. We cannot avoid consideration of this alternative, because it would ‘save’ (40), which has been one of the strongest sources of support for the hypothesis that phonetics largely determines foot shape.

3.7. A trochaic alternative

If the analysis of Paumari stress just presented is correct, then, among other things, it follows that (40) must be abandoned or reinterpreted as a violable OT constraint. But before concluding that (40) is violable or just wrong, however, we must consider a possible alternative analysis, owing to work by Kager (1989) on Tübatulabal. This alternative must be successfully eliminated to establish a warrant for our rejection of (40). Consider first the Tübatulabal words in (41) and (42) (ultimately from Voegelin (1935) but taken here from Hayes (1995:264)). The basic facts are that (i) final syllables bear primary stress; (ii) heavy syllables (CV:) are stressed; (iii) every other light syllable before a heavy syllable is stressed. Crowhurst (1991) proposes to analyze these facts in terms of right-to-left iambs (' = secondary stress; '' = primary stress):

If Crowhurst is correct, then Tübatulabal violates the Iambic/Trochaic Law. Iambic parses of (41) and (42) are given in (43):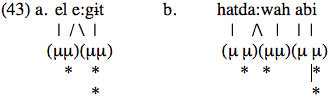

The parses in (43) are quite similar to parses suggested for Suruwahá in Everett (1996). If correct, then once again the Iambic/Trochaic Law is violated. However, Kager (1989) makes a very ingenious proposal to save the Iambic/Trochaic Law, cited in Hayes (1995:264). Kager analyzes Tübatulabal as a trochaic system, avoiding any violation. He does this by top-down parsing, in which the final syllable is stressed first, as a degenerate foot, and then the rest of the word is parsed. This is ingenious because prior to Kager’s suggestion, most analyses were based on bottom-up parsing (and most still are). Hayes (ibid.) summarizes Kager’s proposal as in (44):

If Kager is right in his reanalysis, then the forms in (43) are incorrect. The correct parse for (43a) is given in (45):

But this counterproposal cannot rescue the Iambic/Trochaic Law from the Paumari facts because primary stress in Paumari is on the antepenult in polyvocalic words and cannot, therefore, be accounted for by End Rule Right. So far as I have been able to determine, however, Paumari is the only iambic stress system reported on with antepenultimate stress. This unique aspect of its primary stress makes it a severe counterexample, as we have just seen, for all attempts at reanalysis.

Since Kager’s End Rule Right analysis is the only viable alternative to my iambic analysis of Paumari, and since Kager’s analysis fails to account for Paumari, I conclude that Paumari does indeed violate the Iambic/Trochaic Law. Therefore, it is important to consider the implications of Paumari for prosodic theory.

4. Consequences for foot theory

4.1. The Iambic/Trochaic Law

Kager’s work on iambicity has convinced me, like most phonologists, that there is something important about the proposed Iambic/Trochaic Law. But at the same time the facts of Paumari require us to abandon this as a ‘law’. In OT, this simply means that this ‘law’ is a violable constraint (rather than, say, part of Gen(erate), the function which houses inviolable constraints in OT). If nothing else had ever been said about (40) in modern phonological theory, we could simply leave it at this.

But when I say we ‘simply’ reinterpret this as a violable constraint, I refer to the mechanics of the theory. In actuality, the consequences for a good deal of work in the theory are severe. Several recent works have attempted to derive the Iambic/Trochaic law from the interplay of other constraints in OT. All of these works begin with the assumption that this law is in a sense inviolable, that is, that the empirical generalizations upon which it is based are inviolable. Yet if I am correct here, there is nothing to derive. If the law is merely a violable constraint, there is not much else to say about it. This would have serious implications, however, for some recent work. Perhaps the two major attempts to derive this ‘law’ in Optimality Theory are Eisner (1997) and van de Vijver (1998). Since their factual assumptions about iambicity are nearly identical, I will consider only van de Vijver’s (van der Vijver) project.

van de Vijver claims that “... iambs (rightheaded feet) have four properties which are remarkable and which are not explained by any current metrical theory.” The properties are:

Paumari clearly violates (46a)-(46c). Therefore, these points are false. But if (46a)-(46c) are false, then (46d) becomes irrelevant. There does not seem to be any ‘wiggle room’ here. van de Vijver’s research on iambs, representative of a good deal of recent received opinion about foot structure (tracing back, of course, to the massively influential work by Hayes (1995) and, to a lesser degree, the various research reports by Kager cited in this paper) is in a crucial aspect misguided (see also Eisner (1997:1) also for a nearly identical list of assumptions about iambs, equally falsified by the conclusions of this paper).

But is it fair to throw out otherwise well-developed proposals by e.g. van der Vijver and Eisner based on a single language? Of course it is. As Ladefoged and Everett (1996) conclude, there is no principled way to avoid the implications of data merely because they are ‘rare’ or ‘exotic’, both of which all too often merely describe our sampling techniques rather than the actual distribution of properties of natural language. So, again, the results of this research on Paumari is quite relevant to theories of foot structure in modern phonology. A prudent response to the problems the Paumari data raise, it seems to me, is to hesitate to rush into the proposing of laws and universals of prosody based on our quite impoverished state of knowledge about prosody.

Before concluding this section, there is another theoretical implication of Paumari for the Iambic/Trochaic law which must be addressed. This implication is in some ways more significant than the theory-internal implications we have just considered.

4.2. The phonetic underdetermination of foot structure

The consequences of interpreting (40) as a violable constraint of some form extend beyond the architecture of Optimality Theory. They affect our very understanding of the phonetics-phonology interface. Consider the following remarks from Hayes (1995:70ff), where he argues that the Iambic/Trochaic law is the linguistic formalisation of “... a purely rhythmic principle.” He proceeds to discuss a number of extralinguistic factors to support the idea that the Iambic/Trochaic law in (40) reflects natural perceptual principles of human cognition. He begins by focussing on relatively recent experimental work by Rice (1992) (replicating earlier experiments by other researchers). Hayes notes that this experiment shows the following ‘usual results’: “... in the case of intensity contrast, the preferred grouping is with the most prominent element first ... In the case of durational contrast, the preferred grouping is with the most prominent element last...” Hayes (p80) also discusses research on musicians’ perceptions and notes that these too seem to confirm the perceptual preferences Rice’s work corroborates. This is an interesting result by any standards. And yet we know that not all phonology is driven by phonetics, just as not all syntax is driven by information structure or semantics. What in effect has happened is this, it seems to me. Hayes searched the literature on prosody about as well as anyone ever has and was unable to find clear examples of nonlength-based iambic systems. In addition to this, he was aware of the perceptual research. It is only natural, therefore, that he would propose that the Iambic/Trochaic law as a universal of human cognition, unlikely to be violated in human languages. If that result could stand, then we would have a significant amount of theory of foot structure motivated functionally, externally to linguistics in part arising from phonetics in other aspects (what is easier to hear). But the Paumari facts eliminate this strong interpretation of the Iambic/Trochaic law as determined by phonetics. This means that foot structure (like so much else in grammar, after all) is partly independent of extralinguistic considerations and is a genuine part of grammar. And this is an important result from the Paumari research and the violability of the Iambic/Trochaic Law.

4.3. Foot structure

4.3.1. Introduction

In addition to leading us to abandon (40) as a law, Paumari’s prosody has other significant implications for the theory of foot structure. These implications concern the distribution of degenerate feet and the relationship between degenerate feet and WdMin.

According to Hayes (1995:87), degenerate feet are constrained severely, per (47):

Hayes suggests that (47) might be supplemented by (48):

Hayes is concerned with the distribution of degenerate feet for various reasons. Especially important is the perceived correlation between foot size and word size, apparently enabling the latter to be derived from the former (p. 88): “... a ban on degenerate feet makes predictions about possible word shapes. In particular, assuming that every phonological word must contain at least one foot, and that there are no degenerate feet, then there can be no degenerate-size words.” In this quote, Hayes is in effect proposing the implicational universal in (49):

This implication seems correct, so long as it is restricted to prosodic words, as Hayes intends. However, later in the text (p. 95), Hayes makes it clear that he in fact has a stronger implicational relationship in mind, namely: “... degenerate feet will only occur in strong position, only at the (right/left) edge of the word where footing is (left-to-right/right-to-left), and only in languages that allow degenerate-size monosyllables.” (emphasis mine, DLE)

The emphasized portion of this quote is incorrect, if the analysis above is right. Paumari lacks degenerate words, but it allows degenerate feet. Moreover, its degenerate feet are neither restricted to “strong position”, i.e. to where primary stress falls (see (47) and Tableau 4 above), nor are they completely unrestricted; i.e. they do not fall under Non-prohibition in (48) above.

Hayes (1995:100) argues that apparent cases of degenerate feet in weak positions are likely to turn out in reality to result from lengthening. His claim would be that in a word, like arabo ‘land’, in Tableau 1, the initial a is lengthened, in effect producing a binary foot in word-initial position, as in (50):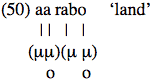

But phonologically relevant lengthening simply does not occur in Paumari. For example, consider how my analysis would represent the word, biakavaka'oahivini ‘to close’ (see Everett (1998) for other examples of Syllable Integrity violations, i.e. dividing syllables between feet):

The representation in (51) predicts that all syllables are equal in length. Even with stress, this is still largely true for most nonfinal syllables and is roughly true for final syllables. The length produced by stress is real, but usually subtle. If the stressed constituents were phonologically long, however, the representation would have to modified as in (52a) or (52b). If we accept my proposal that diphthongs are monomoraic then, with the hypothesized added length, we have (52a):

But (52a) is falsified by the simple fact that stressed vowels are never twice as long as diphthongs. If, on the other hand, we assume that diphthongs are bimoraic, as in (52b), we create a different problem. Consider the penultimate and initial feet in (52b). Both violate the Iambic/Trochaic law because they contain no length contrasts, yet still have final prominence. Since they are of equal length, if we divided them instead into separate feet we would wrongly predict that both feet would be stressed or, alternatively have to appeal to some sort of clash avoidance (the effect of which would be to duplicate the effects of the feet in (51)). Such moves represents a significant and otherwise unwarranted complication of the grammar of Paumari (and they would make it quite unlike any other Arawan language, prosodically; see Everett (1995)). So, we must reject Hayes’ claims regarding degenerate feet.^[3] Let us turn now, though, to consider another influential hypothesis on degenerate feet.

4.3.2. Degenerate Feet, WdMin, and Catalexis

Hayes, as we have just seen, not only predicts that degenerate feet will be restricted to strong positions, he also predicts a very tight correlation between minimal word size and degenerate feet. However, his predictions in this regard fail. But there is another proposal to maintain the relationship between degenerate feet and minimal word size which avoids the problems faced by Hayes’s proposal, so we must consider that proposal as well before closing the present section.

Kiparsky (1991) and Kager (1995a) propose a different, quite ingenious, account of degenerate feet, based on a notion Kiparsky labels Catalexis. According to Kager (1995b, p447), Catalexis is based on the idea that final stresses on so-called degenerate feet are under “grammatical control” and not merely phonetic facts, as in Hayes’ account. Kager (1995n, 447) claims that this allows him and Kiparsky to capture the fact that “... in rightward trochaic systems, the presence of degenerate-size words correlates with the presence of final stresses in odd-numbered words.”

Catalexis claims in effect that there are no degenerate feet, period, and that where there appear to be degenerate feet, there is in reality a “segmentally empty metrical position at the right edge of the word, i.e. essentially the logical counterpart of extrametricality.” (Kager 1995b:447).

In words with odd-numbers of moras, the catalectic mora is footed. It is left unfooted, however, in words of even numbers of moras. It makes the prediction in (53):

Although this correlation is irrelevant in Paumari, Catalexis might nonetheless still be argued to hold, even if vacuously. However, it must be rejected because because of another implication that Kager (1995:447) draws from it, namely, that it is “... predicted that catalectic languages have no word minimum.” But as we have seen, there is a minimal word constraint in Paumari, thus vitiating the Catalexis analysis.

4.3.3. Degenerate Feet and FtBin

As Everett (1990, 1994, 1996), Hewitt (1994), Crowhurst and Hewitt (1997), Downing (1998), and Green and Kenstowicz (1995) have pointed out, the standard statement constraint on foot size, Prince & Smolensky’s (1993:47) must be reformulated. Prince and Smolensky propose FtBin, repeated here:

The reason (54) must be reformulated is that it cannot account for crosslinguistic variation in the ‘repairs’ to violations of it (see the references cited). I will therefore follow proposals of the work cited and reformulate (54) as (55) and (56):

These will operate as follows. Consider the word arabo ‘land’ once again, relative to the constraints in Tableau 8: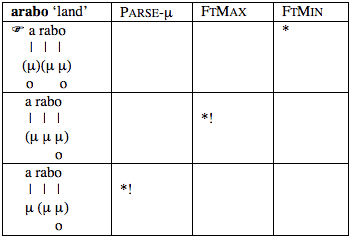

Tableau 8

Since degenerate feet do coexist with a word minimality constraint in Paumari, we must conclude that previous hypotheses on the correlation between minimal foot and word size are incorrect. On the other hand, we can still maintain the weak correlation between the two given in (57), already established above:

This hypothesis bears further investigation, but it seems right. It certainly seems clearly superior to the alternative, and widespread, view in (58), in light of the Paumari facts:

(58) Word Minimality and Foot Binarity: The minimal word is no smaller than the smallest (i.e. binary) foot.

With the conclusion of this discussion of constraints on foot shape, we turn to consider constraints on prosodic words. That is, as stated from the outset...

5. Conclusion

From the analysis of Paumari presented above, we have drawn the following, I believe significant, conclusions for phonological theory. First, we have seen that the Iambic/Trochaic Law does not hold universally and, therefore, can at most be interpreted as a violable constraint in prosodic theory. Abandoning this law, however, severely impacts research represented by van de Vijver (1998), Kager (1989, 1993), and Eisner (1997) which assume that it is universal and propose a series of innovations to Optimality Theory to account for this universality. Since the purported universality of this law has been shown to be spurious (which is what it means to say that it is violable, barring any other evidence for it) the results of such research programs must be largely abandoned. A second major result of this research is the finding that the interesting phonetic and perceptual bases for the Iambic/Trochaic Law noticed by Hayes (1995) underdetermine the shape of feet cross-linguistically. That is, foot structure, like other areas of phonology, is underdetermined by phonetics. This is not entirely surprising, but it does demonstrate a domain about which many had though otherwise. From these results, we also reached the following, ancillary conclusions: (i) The minimal word size of a given language is linked to the shape of well-formed feet, not merely minimal feet as has been believed by most phonologists until now; (ii) The distribution of degenerate feet is restricted by the relative ranking of FtMin, FtMax, and Parsem/s, and not by Hayes’s (1995) proposals on weak vs. strong positions; (iii) Paumari provides additional evidence that the so-called FtBin constraint must be decomposed into the constraints FtMin and FtMax; (iv) There is an urgent need for more fieldwork (see Everett (2001)), shown here by the number of theoretical proposals in need of modication or abandonment as the result of the study of a single heretofore unstudied language.

References

Blevins, Juliette. 2003. The independent nature of phonotactic constraints: an alternative to syllable-based approaches. The syllable in Optimality Theory, ed. by Caroline Féry and Ruben van de Vijver, 375-403. Cambridge: Cambridge University Press.

Buller, Barbara, Ernest Buller, and Daniel L. Everett. 1993. Stress placement, syllable structure, and minimality in Banawá. The International Journal of American Linguistics, 59.280-293. doi:10.1086/466199

Chapman, Shirley, and Desmond Derbyshire. 1991. Paumari. Handbook of Amazonian languages III, ed. by Desmond Derbyshire and Geoffrey K. Pullum, 161-352. Berlin: Mouton de Gruyter.

Chapman, Shirley and Meinke Salzer. 1999. Paumari dictionary. Porto Velho, Brazil: SIL International and http://www.sil.org/americas/brazil/englhome.htm.

Crowhurst, Megan. 1991. Demorification in Tübatulabal: evidence from initial reduplication and stress. Northeastern Linguistics Society 21.49-63.

Crowhurst, Megan and Mark Hewitt. 1997. Directional footing, degeneracy, and alignment. Northeastern Linguistics Society 25.47-61.

Dixon, R.M.W. 2000. Arawa. The Amazonian languages, ed. by R.M.W. Dixon and Alexandra Y. Aikhenvald, 292-306. Cambridge: Cambridge University Press.

Downing, Laura. 1998. On the prosodic misalignment of onsetless syllables, Natural Language and Linguistic Theory 16.1-52.

Eisner, Jason. 1997. FootForm decomposed: using primitive constraints in Optimality Theory. http://ruccs.rutgers.edu/roa.html, paper ID = ROA-205-07972.

Everett, Daniel L. 1990 Extraprosodicity and minimality in Kamã and Banawá. Pitt Working Papers in Linguistics 1.17-41. Department of Linguistics, University of Pittsburgh.

Everett, Daniel L. 1995. Sistemas prosódicas da família Arawá. Estudos fonológicos de línguas indígenas brasileiras, ed. by Leo Wetzels, 297-339. Rio de Janeiro: Editora da Universidade Federal do Rio de Janeiro.

Everett, Daniel L. 1996. Prosodic levels and constraints in Banawá and Suruwahá. http://ruccs.rutgers.edu/roa.html, paper ID = ROA-121-0496.

Everett, Daniel L. 1998. Syllable Integrity. West Coast Conference on Formal Linguistics 16.177-190. Chicago: University of Chicago Press.

Everett, Daniel L. 2001. Monolingual field research. Linguistic fieldwork, ed. by Martha Ratliff and Paul Newman. Cambridge: Cambridge University Press.

Ewen, Colin and Harry van der Hulst. 2001. The phonological structure of words: an introduction. Cambridge: Cambridge University Press.

Gerfen, Chip. 2001. A critical view of licensing by cue: codas and obstruents in Eastern Andalusian Spanish. Segmental phonology in Optimality Theory, ed. by Linda Lombardi, 183-205. Cambridge: Cambridge University Press.

Green, Thomas and Michael Kenstowicz. 1995. The lapse constraint. http://ruccs.rutgers.edu/roa.html, paper ID = ROA-121-0496.

Hayes, Bruce. 1995. Metrical stress theory: principles and case studies. Chicago: University of Chicago Press.

Hewitt, Mark. 1994. Deconstructing foot binarity in Koniag Alutiig. ms. University of British Columbia and http://ruccs.rutgers.edu/roa.html, paper ID = ROA -12-0000.

Kager, René. 1989. A metrical theory of stress and destressing in English and Dutch. Dordrecht: Foris.

Kager, René. 1993. Alternatives to the iambic-trochaic law. Natural Language and Linguistic Theory 11.381-432. doi:10.1007/bf00993165

Kager, René. 1995a. Consequences of catalexis. Leiden in Last: HIL Phonology Papers I, ed. by Harry van der Hulst and Jeroen van de Weijer, 269-298. The Hague: Holland Academic Graphics.

Kager, René. 1995b. Review of Metrical Stress Theory: Principles and Case Studies, by Bruce Hayes. Phonology 12.437-464.

Kager, René. 1997. Rhythmic vowel deletion in Optimality Theory. Derivations and constraints in phonology, ed. by Iggy Roca, 463-499. New York: Oxford University Press.

Kiparsky, Paul. 1991. Catalexis. Stanford: Stanford University & Wissenschaftskolleg zu Berlin, ms.

Ladefoged, Jenny, Peter Ladefoged, and Daniel L. Everett. 1997. Phonetic structures of Banawá, an endangered language. Phonetica 54: 94-111. doi:10.1159/000262213

Ladefoged, Peter and Daniel L. Everett. 1996. On the problem of phonetic rarities. Language 72.794-800. doi:10.2307/416103

Prince, Alan & Paul Smolensky. 1993. Optimality theory: constraint interaction in generative grammar. Rutgers: Technical Reports of the Rutgers University Center for Cognitive Science.

Rice, Curtis. 1992. Binarity and ternarity in metrical theory: parametric extensions. Austin: University of Texas dissertation.

Steriade, Donca. 1997. Phonetics in phonology: the case of laryngeal neutralization. Los Angeles: University of California, ms.

van de Vijver, Ruben. 1998. The iambic issue: iambs as a result of constraint interactions. The Hague: Holland Academic Graphics.

Voegelin, Charles. 1935. Tübatulabal grammar. University of California Publications in American Archaeology and Ethnology, Vol. 34. 55-189. Berkeley: University of California Press.

^[1]I would like to thank Zoe Butterfint for help with the phonetic analysis of many of the examples in this paper. Although the spectrograms were not ultimately included here, her help in preparing them and discussing them with me was extremely useful in preparing this study. Thanks to many Paumari people who gave generously of their time to help me learn about their stress system. Thanks also to Donna Popky who helped with data-collection. I especially want to thank Shirley Chapman for discussing the examples and analysis here in considerable detail. Although she has not yet agreed with this analysis, without her pioneering work among the Paumari, the research here would not have been possible.

^[2] The starred examples in (6) are intended to show that iambic feet are oriented towards the right, rather than the left. Even-numbered words, when viewed alongside the odd-numbered words, render an alternative analysis in terms of trochees opaque at best.

^[3] Moreover, the entire claim of lengthening as a universal of iambic systems is rationalistic, not empirical. It is not empirical because there are far too few phonetic studies of prosodic systems to hazard any such guesses as to what is likely to be universally true of this or that stress system. Much, much more phonetic work is needed. I believe that more fieldwork will turn up many counterexamples to (13), in all of its aspects.